Page 92 - Rollingbearings

B.3 Bearing size
Calculating bearing life with required under the load condition P , and N
1
variable operating is the expected number of revolutions for the
conditions, luctuating load completion of all variable loading cycles,
then the cycle fraction U = N /N is used by
1
1
In some applications – for example, industrial the load condition P , which has a calculated
1
gearboxes, vehicle transmissions or wind- life of L 10m1 . Under variable operating condi-
mills – the operating conditions, such as the tions, bearing life can be rated using
magnitude and direction of loads, speeds,
1
temperatures and lubrication conditions, are L 10m = —————————
U 1 U 2 U 3
continually changing. In these types of appli- — — — ...
+
+
+
L
L
cations, bearing life cannot be calculated L 10m1 10m2 10m3
without irst reducing the load spectrum or
duty cycle of the application to a limited where
number of simpliied load cases (diagram 3). L 10m = SKF rating life (at 90%
For continuously changing loads, each reliability) [million
different load level can be accumulated and revolutions]
the load spectrum reduced to a histogram L 10m1 , L 10m2 , ... = SKF rating lives (at 90%
plotting constant-load blocks. Each block reliability) under constant
should characterize a given percentage or conditions 1, 2, ... [million
time-fraction during operation. Heavy and revolutions]
normal loads consume bearing life at a U , U , ... = life cycle fraction under
1
2
faster rate than light loads. Therefore, it is the conditions 1, 2, ...
important to have peak loads well repre- U + U + ... U = 1
2
1
n
sented in the load diagram, even if the
occurrence of these loads is relatively rare The use of this calculation method is well
and of relatively short duration. suited for application conditions of varying
Within each duty interval, the bearing load load level and varying speed with known
and operating conditions can be averaged to time fractions.
a representative, constant value. The number
of operating hours or revolutions expected
from each duty interval, showing the life
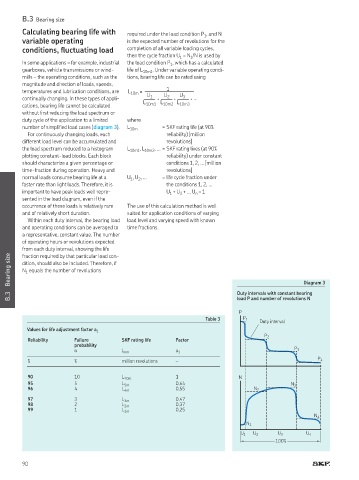
Bearing size dition, should also be included. Therefore, if Diagram 3
fraction required by that particular load con-
N equals the number of revolutions
1

B.3 Duty intervals with constant bearing
load P and number of revolutions N

P
Table 3 P 1
Duty interval
Values for life adjustment factor a 1
P 2
Reliability Failure SKF rating life Factor
probability
n L nm a 1 P 3
% % million revolutions – P 4

90 10 L 10m 1 N
95 5 L 5m 0,64 N 3
96 4 L 4m 0,55 N 2
97 3 L 3m 0,47
98 2 L 2m 0,37
99 1 L 1m 0,25
N 4
N 1
U 1 U 2 U 3 U 4
100%

90